- Mole And Avogadro's Number Explained
- The Mole And Avogadro's Number Key
- Define Mole And Avogadro's Number
Lab 17: The Mole and Avogadro’s Number
Avogadro’s Constant-The number of elementary entities in one mole of any substance is 6.022×10 23. The idea of such a number was first conceived by Italian scientist Amedo Avogadro. Although he never determined this number, in his honour, this number, along with its unit (per mole) is called Avogadro’s Constant. Avogadro's Number:Counting Atoms. Owing to their tiny size, atoms and molecules cannot be counted by direct observation. But much as we do when 'counting' beans in a jar, we can estimate the number of particles in a sample of an element or compound if we have some idea of the volume occupied by each particle and the volume of the container.
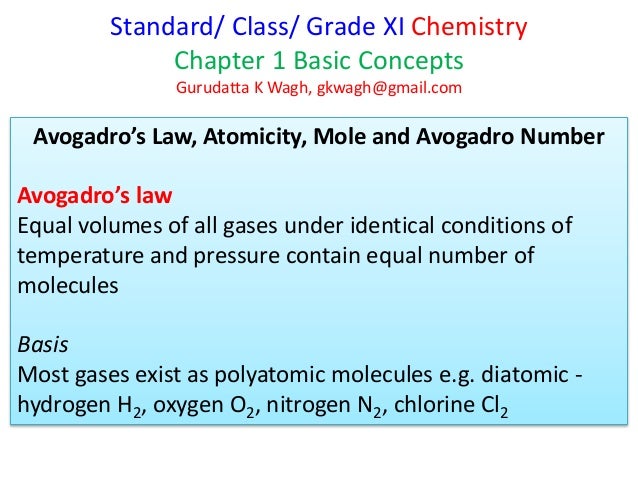
Introduction
“Avocados” number: How many avocados and artichokes are there in a mole?
A recipe calls for two avocados and two artichokes. Would you say that equal amounts of avocados and artichokes are used? The answer to that question depends on how you define “the same amount”. If you consider the quantity, yes, there are two of each. What if instead the recipe told you to use 250 grams of artichokes and avocados? There might be one and a half avocados used for every arti‐choke—would you call this the same amount as well?
One way to solve this problem would be to ask for more specific instructions. The phrase “the same amount” is being used to describe quantity (number) in one instance and mass in another. Similar situations come up in chemistry. If you are supposed to put the same mass of two different substances into a beaker, all you would have to do is weigh equal amounts using a scale. Things become more complicated, however, when the ratio of the total number of molecules or atoms is important in an experiment—you might know the number of molecules in the reactants and products, but not the actual masses. How would you measure an exact number of NaCl molecules?
The mole is an important unit in chemistry that you will use often. The modern definition of the mole is based around the carbon‐12 (12C) atom: 12 grams of 12C is exactly one mole of substance. It turns out that 1 gram of 12C has approximately 6.02 x 1023 individual atoms—a value called Avogadro’s Number after the chemist Amedeo Avogadro. The mole is a similar concept to a dozen: one mole of a substance will always have 6.02 x 1023 atoms or molecules.
Atomic weight is a measurement of the mass of each element, and can be easily found on most periodic tables. Atoms that have a large number of protons and neutrons have more total mass than atoms with only a few protons and neutrons, and this difference is reflected by the atomic weight. Conveniently, the mole and atomic weight are defined so that one mole of a substance will have a mass equal to the atomic weight of that substance in grams. This number is called molar mass. For instance, the atomic weight of potassium (K) is 39.098. This means that one mole of potassium will have a mass equal to 39.098 grams. Calcium (Ca) is a larger atom than potassium, and has an atomic weight of 40.078. One mole of calcium will have the same number of atoms as a mole of potassium, but the Calcium will weigh more due to its larger atomic weight.
See the next lab for more detail on atomic weight and atomic mass.
So how do you measure 1 mole of NaCl? We know that this molecule is made up of one sodium ion and one chlorine ion (chloride). Looking up sodium (Na) on the Periodic Table tells you its atomic weight is 22.99, meaning it has a molar mass of 22.99 g/mol. Chlorine, meanwhile, has a molar mass of 35.45 g/mol. Since one mole of sodium chloride consists of one mole sodium ions and one mole chlorine ions, we can add these together to find the molecular mass of NaCl.
Figure 1: You can think of avocados and artichokes in this example as two different types of molecule—each with a different
molar mass.
Concepts to explore:
Understand the importance of Avogadro’s Number
Approximate the value of Avogadro’s Number 180
What if we weighed out 1.00 grams of NaCl—how many molecules is this? We use the molar mass of NaCl, which we already
know from above, to convert from mass to a number of moles. We can then use the fact that there are 6.02 x 1023 molecules in
a mole to find the number of molecules NaCl in one gram:
Notice how the molar mass is inverted in the second term. The value is the same, but we “flip” the fraction so that the gram
units cancel. You can go through and cross out the units that cancel to verify that the resulting units are molecules. You can
use similar calculations to convert between mass, moles, and the number of molecules fairly easily.
Through this lab procedure, we will determine the experimental value for Avogadro’s number. You will float cinnamon, evenly
distributed, on the surface of water in a Petri dish. The dishwashing liquid you will use in this Lab is about 1% sodium stearate,
and a solution with a known concentration of the liquid will be dropped onto the water. The sodium stearate molecules will
form a single layer and spread out, pushing the cinnamon toward the edges of the Petri dish, allowing the surface area to be
determined. We will assume that each molecule takes up 0.210 nm2 of surface area, and that there is no space between the
molecules.
23
22
1 mol NaCl 6.02 10 molecules
1.00 g NaCl 1.03 10 molecules NaCl
58.44 g NaCl 1 mol NaCl
1 Na = 22.99 g/mol
+ 1 Cl = 35.45 g/mol
58.44 g/mol of NaCl
This is how to determine molar mass of a compound.
1 mol NaCl x 58.44 g NaCl = 58.44 g NaCl
1 mol NaCl
Pre‐lab Questions
1. How many grams of H2O do you need to weigh out to have 1 mole of H2O?
2. How many molecules of water are there in one mole of H2O?
3. How many moles of H2O are there in 1.0 g of H2O?
4. How many molecules of H2O are there in 1.0 g of H2O?
Lab 17: The Mole and Avogadro’s Number
Experiment: Avogadro’s Number
Procedure
Part 1: Preparing the Sodium Stearate Solution
1. Measure exactly 1.50 mL of dishwashing liquid into a 10 mL graduated cylinder.
2. Fill a wash bottle with distilled water. Gently rinse the 1.50 mL of dishwashing liquid with distilled water and
pour it into a 100 mL graduated cylinder. Rinse the 10 mL graduated cylinder several times to make sure all
the dishwashing liquid has been transferred to the 100 mL graduated cylinder. HINT: Try not to create suds.
3. Add enough additional distilled water to get to the 100.0 mL.
4. Gently stir the solution with a stirring rod until it is mixed well.
Part 2: Calibrating a Dropper
1. Fill a 50 mL beaker half full with distilled water. Use a pipette to fill a 10 mL graduated cylinder to 1.00 mL
with water. HINT: Make sure the 10 mL graduated cylinder is clean of dishwashing liquid.
2. Next, draw up water from the 50 mL beaker into the pipette. Add water dropwise into the graduated cylin‐
der. Hold the pipette consistently at a 45o angle and drop at a rate of about one drop per second. Count the
drops it takes to reach the 2.00 mL mark. HINT: It should take about 25 drops. If you feel that your measure‐
ment is incorrect, repeat until you achieve consistent readings.
3. Record in the Data section the number of the drops it takes to add 1 mL water to the graduated cylinder.
4. Repeat calibration for a second trial, and record the number of drops in the Data section. Average the two
results.
Mole And Avogadro's Number Explained
Part 3: Calculating the Number of Molecules
1. Rinse and then fill a petri dish with 20 mL distilled water. Allow the water to settle and remain motionless.
Materials
Safety Equipment: Safety goggles, gloves
| Ground cinnamon | 10 mL graduated cylinder |
| Dishwashing liquid | Stirring rod |
| Dropper (pipette) | 50 mL beaker |
| Petri dish (bottom) | Wash bottle |
| Ruler | Distilled water* |
| 100 mL graduated cylinder | *You must provide |
2. Lightly sprinkle cinnamon onto the surface of the water in the Petri dish. HINT: Add just enough to barely
cover the water.
3. Draw up the dishwashing liquid solution with the calibrated pipette. Hold the pipette at a 45o angle about 1
inch above the center of the Petri dish. Slowly deliver one drop of the solution. HINT: A clear circle should
form, spreading the cinnamon outward.
4. Quickly use a ruler to measure the diameter of the cleared circle in cm.
5. Record the diameter in the Data section. Wash out the Petri dish.
Data
Part 2: Calibrating a Dropper
1. The number of drops in 1 mL water (drops used to move from the 1.00 mL to 2.00 mL mark):
Trial 1: Trial 2:
2. The number of drops on average per one milliliter:
Part 3: Calculating the Number of Molecules
1. The diameter of the circle formed (cm):
Calculations
1. Calculate the surface area of the circle formed ( πd2 /4 ) :
2. Calculate the number of molecules on the top layer. We must convert the surface area in centimeters
squared to nanometers squared and then multiply that by the surface area of a sodium stearate molecule.
Convert the surface area of the circle formed (#1) to molecules per layer:
Surface area =
| cm2 | 1 m2 | 1 x 1018 nm2 | 1 molecule |
| Top layer SA (Question 1) | 10,000 cm2 | 1 m2 | 0.210 nm2 |
=
molecules
top layer

Lab 17: The Mole and Avogadro’s Number
3. Calculate the concentration of grams of sodium stearate per milliliter of diluted solution. To do this, multiply the
concentration of sodium stearate in the dishwashing liquid by the dilution of the solution (1.50 mL dishwashing
liquid per 100 mL solution).
4. Calculate the number of moles of sodium stearate in a single layer. To do this, first take the number of drops used
to achieve the monolayer (1 drop) and convert it to mL using the calibrated number of drops per mL. Then multi‐
ply the number of grams of sodium stearate per milliliter of solution. Finally, convert to moles through the molar
mass of sodium stearate. HINT: The molar mass of sodium stearate is 296.4 g/mol.

| 1 g sodium stearate | 1.50 mL dish liquid |
| 100 mL dish liquid | 100 mL diluted solution |
= g /ml
= mol / top layer
5. Finally, we can calculate the Avogadro’s number through the comparison of molecules of sodium stearate in the
top single layer to the moles of sodium stearate in the monolayer.
Avogadro’s number (experimental) =
# molecules / top layer (#2)
# moles / top layer (#4)
molecules
= mole

| 1 drop (added to dish) | 1 mL dish liquid solution | g sodium stearate (from #3 calculation) | 1 mol |
| top layer | drops (avg # calibrated per mL from Data Part 2) | 296.4 g (molar mass of sodium stearate) | 1 mL dish liquid solution |
Post‐lab Questions
1. Why do you think that Avogadro’s number, 6.02 x 1023, was probably not the exact number you obtained?
Was your experimental value close to the actual value (i.e., was your experimental value on the order of 1023
molecules)?
2. How many moles are in 0.289 g of methane (CH4)?
3. How many moles are in 1,000,000,000 molecules of H2 02?
4. What is the mass in grams of 1,000,000,000 (109) molecules of H2O2?
Lab 17: The Mole and Avogadro’s Number
Last Updated on December 15, 2020 by
Related posts:
Mole Concept:
Whether we weigh a substance or measure the volume of a gas, any sample of matter we examine contains particles called molecules which in turn are composed of atoms. Scooby doo and the spooky swamp pc. Thus, we never work with individual atoms or molecules but always with the aggregates of these.
The Mole And Avogadro's Number Key
Now, it is a common practice to count the particles in convenient numbers. For example- we count oranges in dozen, money in rupees, paper sheets by reams and so on. Chemists, therefore, have selected a unit much larger than a single atom or molecule for the purpose of comparison of the amounts of various materials. To a chemist, this unit, termed as a mole, means a definite number of individual unit particles of whatever kind of material is being considered. During the nineteenth century, it was decided by the chemists to take the number of molecules in a sample of oxygen weighing 32 grams as a standard number. A mole, therefore, was defined as the number of oxygen molecules in exactly 32 grams of oxygen. Mole is identified with this number of particles rather than weight. The number determined later to be 6.023 X 1023 is called Avogadro’s number (N). Thus, a mole is Avogadro’s number of chemical units under consideration. Hence, the number of particles present in a mole is 6.023 X 1023. For example, a mole of chlorine atoms includes 6.023 X 1023 atoms of chlorine and a mole of chlorine molecules includes 6.023 X 1023 molecules or 2 X6.023 X 1023 atoms of chlorine because each chlorine molecule contains two atoms.
Significance of a Mole:
The significance of a mole is that it refers to both number (6.023 X 1023) as well as the mass in grams, numerically equal to the molecular, atomic or ionic mass in atomic mass units. For example-
(1) One mole of oxygen signifies-
- 6.023 X 1023 molecules of oxygen or 2 X6.023 X 1023 atoms of oxygen as each oxygen molecule, O2, is diatomic.
- 32 grams of oxygen (because the molecular mass of oxygen is 32 a.m.u).
(2) One mole of water signifies-
Define Mole And Avogadro's Number
- 6.023 X 1023 molecules of water.
- 18.016 or 18 grams of water (because the molecular mass of water is 18.016 or 18 a.m.u).

